This is a rough guide to determine the distance of the horizon based on the observer's height above mean sea level. The screen will work in Metric or Imperial measurements. Enter the height above Sea Level either in Metres or Feet. Press the Calculate button and the distance of the horizon will be displayed in Kilometres when the observer's height is in metres or Miles when the observer height is entered in feet.

The Mathematics behind this Calculation

This calculation should be taken as a guide only as it assumes the earth is a perfect ball 6378137 metres radius without an atmosphere. It also assumes the horizon you are looking at is at sea level. The calculations ignore the atmospheric conditions that will affect the real distance of the visible horizon.

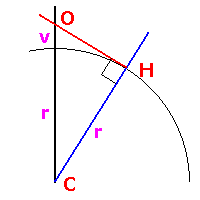
A triangle is formed with the centre of the earth (C) as one point, the horizon point (H) is a right angle and the observer (O) the third corner. Using Pythagoras's theorem we can calculate the distance from the observer to the horizon (OH) knowing CH is the earth's radius (r) and CO is the earth's radius (r) plus observer's height (v) above sea level.

Sitting in a hotel room 10m above sea level a boat on the horizon will be 11.3km away. The reverse is also true, whilst rowing across the Atlantic, the very top of a mountain range 400m high could be seen on your horizon at a distance of 71.4 km assuming the air was clear enough.